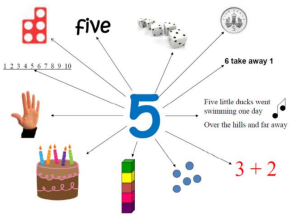
Learning maths is like building a tower; children must have firm foundations and acquire specific building blocks in a certain order. If any of these blocks of understanding are missing, then the tower is shaky and can be toppled at any time with the presentation of new challenges or concepts.
Therefore, at Coleridge, we teach maths differently. Children are not simply rushed through the curriculum, gaining vast, but superficial knowledge that can fall apart whenever they encounter something unfamiliar. Instead, significant time is spent ensuring children have a deep, sustained knowledge of mathematical concepts and that secure building blocks are in place before they are moved on.
Children are also taught to embrace the value of mistakes and false starts; to challenge each other’s thinking and to share what they ‘notice’, without fear of getting something wrong. In doing so, we have fostered ever positive attitudes in children who can think for themselves and persevere in the face of challenges.
To do all this, Coleridge has adopted a mastery approach to teaching maths. As a school, we are immensely proud that over the last few years, we have been able to work very closely with 2 in-school maths experts, both of whom have been trained by the NCETM as Mastery Specialists, to develop expertise in mastery pedagogy and embed excellent practice in our school. During this time, these specialists worked closely with a number of other schools to help them experience, experiment with, and implement ‘teach for mastery’ approaches within their own communities. Our mastery approach produces outstanding results, with a huge majority of our children making expected, or better than expected progress each year.
A Mastery Curriculum at Coleridge
At Coleridge, we have designed and planned our own programme of study in maths, using the National Curriculum, as well as materials produced by the NCETM and White Rose Maths, as a guide.
Taking full control of our curriculum has allowed us to ensure that every unit of works builds on previous learning, both within each year and in subsequent years, and that our core philosophy of maths teaching – that children learn how to think and solve creatively – remains at its heart.
Small Steps of Progression
Lessons do not simply serve to tick off a list of objectives from the national curriculum, one at a time. Instead, these objectives are seen as the end goal, with each unit taking into consideration how children will reach them; what small steps, what skills and knowledge will help the children achieve these goals? As a result, each unit of work is taught over several weeks, with topics being broken down into key skills which are carefully mapped out into a coherent, logical sequence. These small steps of progression allow children plenty of time to grasp and rehearse every concept and master each skill before they move on to the next.
Progression within a lesson also follows this same principle, with each part building on the previous. This allows as many children as possible to keep up with the learning and make sufficient progress.
Representations and Manipulatives
To achieve real mastery in maths – to become true mathematicians who can think mathematically – children need to develop two things: conceptual understanding and procedural fluency.
At Coleridge, a great deal of emphasis is placed on developing the former. The majority of ideas in maths are entirely abstract, generally characterised by a string of symbols that, without any understanding of what they signify, are simply meaningless. In order for children to attach meaning to these abstract ideas, we need to expose them to their structure.
Therefore, to help children construct a mental image of the maths, physical and visual resources are used in nearly all lessons. These are presented alongside abstract representations to allow children to continually make links between them. The teacher may vary which of these – concrete, pictorial or abstract – they focus on in a lesson, but children are exposed to all three, either sequentially or simultaneously. Teachers also ensure there is sufficient variation in the representations and examples that they use, as different representations of the same concept can be used to reveal more of the mathematical structure and in turn, deepen children’s understanding (more on this later).
Manipulatives are a key component in all children’s learning of maths; they are certainly not just reserved for those that are struggling. In fact, at Coleridge, teachers often require children to use manipulatives to prove they have a greater depth of understanding.
However, we strive to ensure that no child becomes entirely dependent on them, relying on them for the how, instead of the why. As soon as they are ready to do so, children are encouraged to visualise the resources instead, using drawings to help them if necessary, before eventually moving on to working wholly in the abstract.
Similar models, representations and resources are used from Reception, right up to Year 6, to ensure children consolidate their understanding and make links between different concepts.
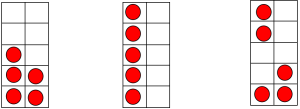
For example, an array will be used from KS1 into the juniors to represent multiplication:
![]()
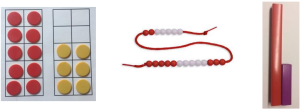
A tens frame, which starts as a fives frame in Reception, will be used in KS1 to represent small, whole numbers, but in upper KS2 to represent decimals:
![]()
Equal attention is given to developing children’s procedural fluency – their ability to use appropriate procedures accurately, flexibly and efficiently to solve a problem. Teachers at Coleridge ensure that children don’t simply learn facts and procedures off by heart, but instead understand the meaning and purpose behind them. In this way, we find that children make suitable and sensible choices when selecting a strategy to solve a problem.
Language
Mathematics requires children to talk like mathematicians, through the use of highly specialised and technical vocabulary. Therefore, teachers place huge emphasis on children being able to share their reasoning rather than just answers. All too often, however, children struggle to articulate even the most simple of thought processes clearly, commonly providing vague responses such as ‘I just knew it’ or ‘I worked it out in my head’. I once even had a child tell me ‘a ladybird’ had given them the answer! For this reason, teachers adopt a number of strategies to aid the development of children’s language, and in turn their reasoning skills:
- Stem sentences are often used alongside mathematical representations to reveal their structure or provide a generalisation. The ‘I say, you say…’ approach, where a stem sentence is first provided by the teacher but then copied by the class, is a way to get children to rehearse these ideas and, over time, make them more available to their own independent thinking.
- Teachers will often model their own thinking out loud to the class.
- Examples of other people’s reasoning will be discussed as a group.
- Relevant mathematical terminology is specifically taught and discussed.
- As well as strengthening conceptual understanding, manipulatives are used to provide a common language to talk about the maths. Teachers will encourage children to use these physical representations to structure their thinking and facilitate their explanations and reasoning.
- Thinking time and partner talk are used in every lesson.
Variation
To ensure that children gain a comprehensive understanding of each concept, teachers provide sufficient variation in the representations and examples that they give. The examples below, for instance, highlight the many ways in which the fraction ¼ can be presented:
![]()
Furthermore, teachers pay careful attention to the order in which they present questions to the children so that important features of a concept or strategy are emphasised.
For example:
120 – 90 = ___
122 – 92 = ___
119 – 89 = ___
Differentiation
A key principle of the mastery approach in maths is that children are taught as a whole class, with each child being given access to the same lesson content. Appropriate support is available for any child who might need it, and there are opportunities to deepen learning even further through the provision of more challenging activities. No assumptions are made before the lesson about which children might need more support, nor which ones will likely move on to the more difficult questions.
Tasks are carefully constructed so that, as children move through them, they become progressively more challenging and an appropriate level of scaffolding is provided at each stage to ensure that more children can access them and deepen their understanding. Open-ended tasks, which all children can access at their own level, are regularly used as well.
Lesson Design
Lessons will commonly be taught using a ‘ping pong’ style approach, so called because the teacher orchestrates a continual back-and-forth dialogue with the children, using questions, short tasks, explanations, demonstrations, and discussions. This enables the teacher to vary the pace and direction of the lesson if necessary, and to continuously monitor the progress of the whole class.
Talk partners and table groups are not fixed and teachers may vary how they organise their class depending on the requirements of the lesson.
Problem Solving and Reasoning
Skills teaching is of course essential, but a curriculum focussed solely on developing competence with number, without opportunities for rich problem solving, is akin to being taught scales on a piano but never learning to play music. Providing children with the skills required to solve mathematical problems in life should therefore be the principal aim of any maths curriculum. It is crucial that, in addition to teaching number skills and number fact, children are exposed to rich mathematical problems in every single lesson.
Therefore, at Coleridge, our children do not learn just how to ‘do maths’; huge emphasis is also placed on teaching them to ‘think mathematically’. Through contextualized problem solving activities and thought-provoking tasks, children work in the same way real mathematicians do, learning skills such as reasoning, conjecturing, generalizing and working systematically. This allows children to experience maths as something that is fundamentally useful, and not just a series of skills that must be learnt in order to pass tests.
Maths in the EYFS
Our EYFS maths curriculum is designed to ensure that, throughout the year, all children develop a secure foundation in the six key areas of early mathematical understanding: pattern, measure, shape and space, cardinality and counting, comparison, and composition. We also ensure our children are exposed to, and feel secure in, the five principles of counting: the one-to-one principle, the stable order principle, the cardinal principle, the abstraction principle, and the order irrelevance principle.
Each week, there is a maths focus in the classroom and children will be able to access the carefully-planned-for activities within the continuous provision to develop their understanding of the week’s focus. These activities might include games in the outdoor provision, craft activities, or role play scenarios. The children will also participate in active and engaging whole class sessions throughout the week, designed to expose them to key mathematical concepts, and illustrate important mathematical language.
In addition to this, teachers ensure that key mathematical learning is drawn out, where possible, from other areas of the curriculum too, such as from key texts they are reading, artists they are studying, or science activities they are discussing. Maths also forms part of the children’s everyday routines, for example, using the ‘Tens Frame’ register, singing maths songs, and counting resources during tidy up time.
We know that developing a mathematical vocabulary in the early years is vital to children being able to access the maths curriculum in Year 1 and beyond. EYFS staff encourage children to use the vocabulary they have been learning in whole class sessions throughout the day in their play. We know that when children use mathematical language in a way that feels relevant to them and their experiences, they are more likely to retain it.
At Coleridge, we believe that children’s early experiences of maths in school should be interesting, fun, challenging and exciting. We want children to spot patterns, ask questions, make suggestions, and to think like mathematicians from the very start.
Catch Up and Keep Up
Teachers at Coleridge have high expectations for all children and firmly believe that every single one can succeed in this subject, that no child ‘just can’t do it’. Lessons are carefully planned to take into account common misconceptions and particularly difficult concepts, ensuring that all children can access the lesson content, either through skilled questioning, supportive scaffolding or appropriate resourcing.
Using various assessment for learning strategies, teachers also have the skills to quickly adapt lessons, as well as sequences of lessons, to address the needs of their children. Additional steps may be added, or the pace of a lesson adjusted, to ensure that they continue to firm up the foundations of their knowledge and understanding, and nurture their love for the subject.
Despite a teacher’s best efforts, however, some children may still struggle with a particular concept. These children are quickly identified and extra support or intervention is put in place to help them keep up.
Coleridge has a team of intervention support staff who are trained to deliver a number of maths interventions:
Becoming First Class at Number
First Class at Number (1 and 2)
Talk for Number
Success at Arithmetic
Number Champions
Marking and Feedback
The most successful form of marking and feedback is that which the children receive instantly. Where possible, therefore, lessons are designed so that immediate feedback to an activity can be given through class discussion. For activities where instant feedback is not possible, children are provided with answer sheets so that they can mark their own work. They are encouraged to do so honestly and overtime, learn how to evaluate their own learning by identifying for themselves where they might need more support.
Teachers will always review the children’s work at the end of a lesson, using it to help inform their planning of the next. A distinction, however, is drawn between errors that arise from misconceptions, and those that are considered accidental mistakes.
RedStart – A Financial Education
Coleridge has partnered up with the charity RedSTART to deliver the ‘Changing the Game’ programme to all children in Years 2 to 6 in the Spring and Summer terms. The programme involves fun and interactive workshops which provide children with a fantastic financial education. We are really excited about what the children will learn and the great life skills it will leave them with. To find out more about this programme, and to see the specific learning objectives for each year group, please see this document or check out the RedStart website.